How do we perceive the existence of the four -dimensional space?Is there really a surface that will not end?

First do the following experiments to feel the magic of Mobius Ring.

Start drawing the line from any point of the Mobius ring with a pen. You will find that you don't have to cross the edge of the Mobius ring, the handwriting will be covered with the entire paper ring, regardless of the front and back, and return to the starting point. There seems to be nothing special, but what if the handwriting is imagined as the route we take? This means that we will go infinitely on the Mobius ring.

The reason for this is because the Mobius ring actually has only one face and is not directed. If you walk along the handwriting, when you return to the original position, you will actually become your own mirror.

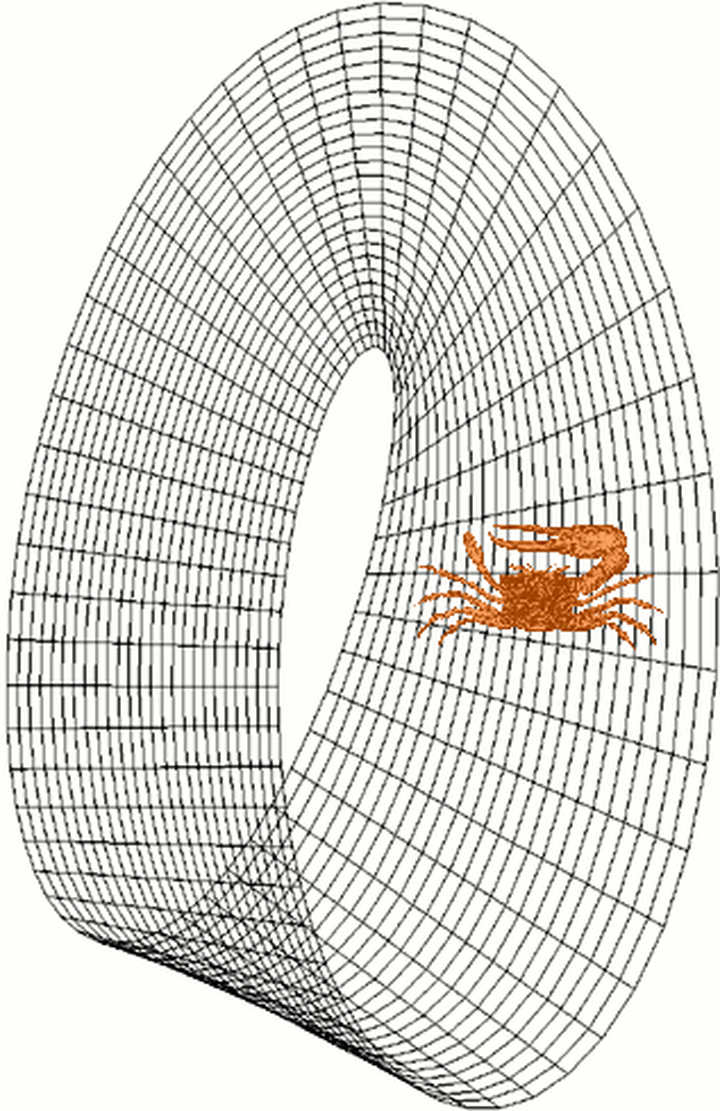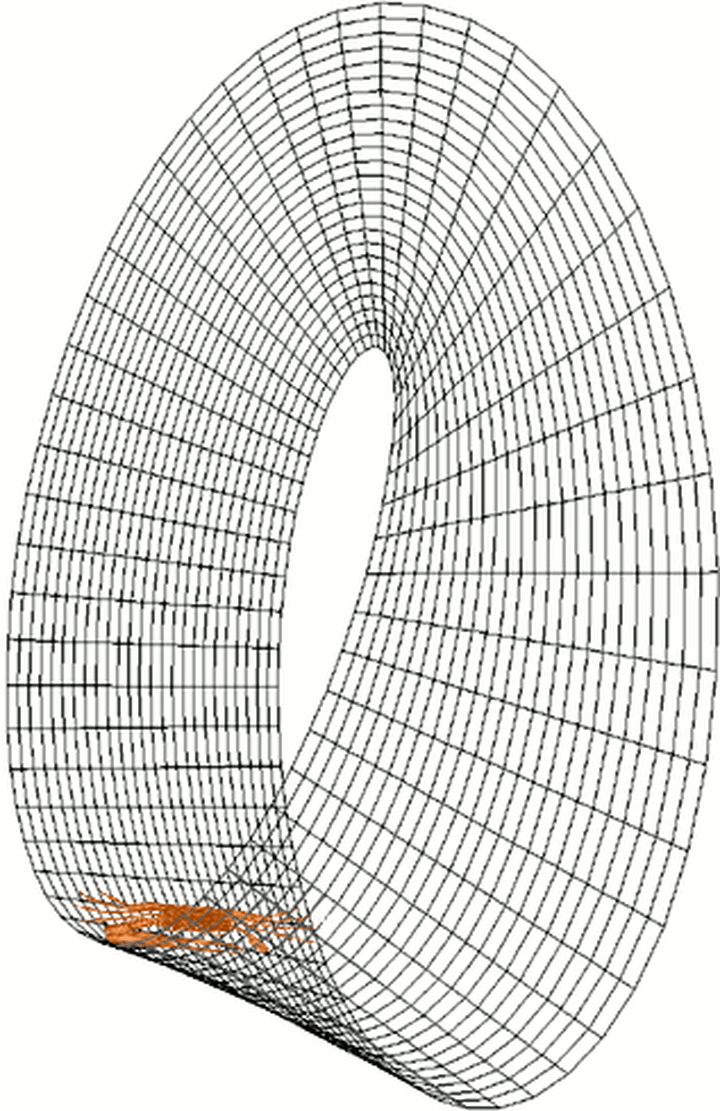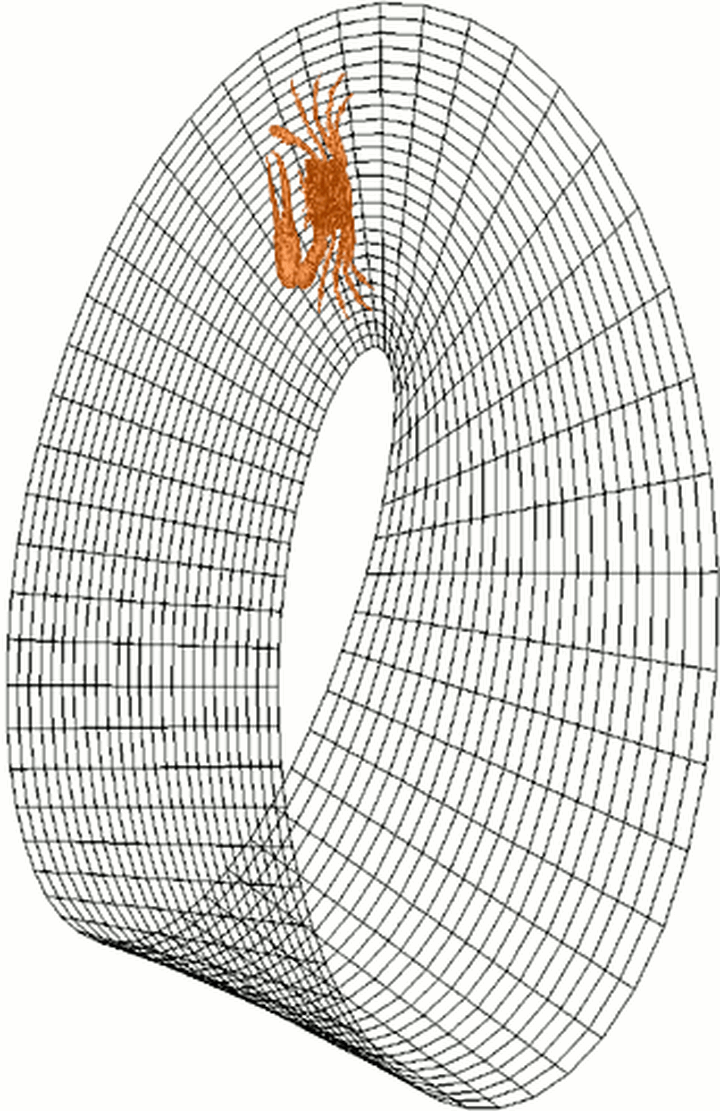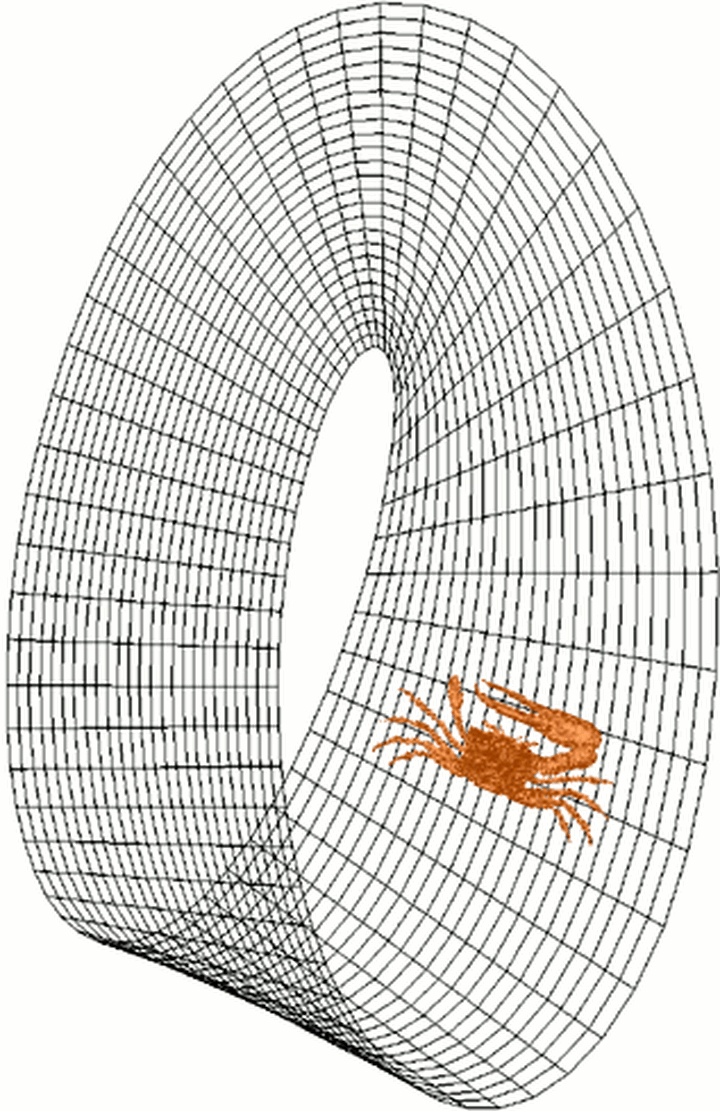
When the crab walks on the Mobius ring and returns its original position, it is its own image. Data source: wikimedia commons

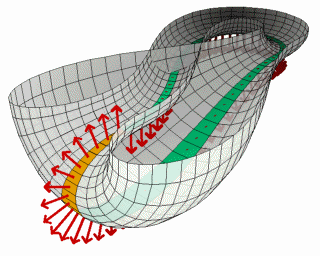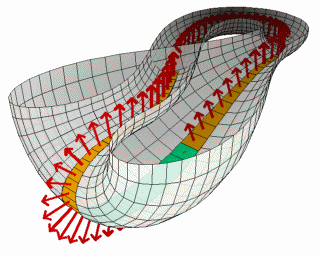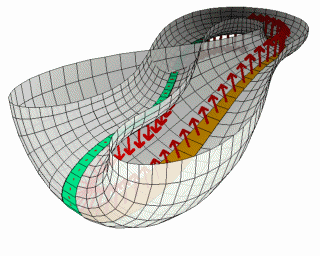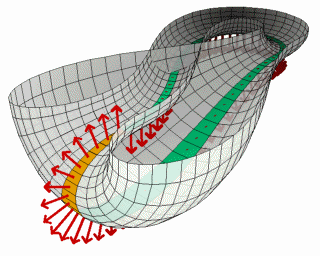
At the same time, we imagine that if a curved surface is extended up and down, and then the Mobius ring is made to distort it one end and then connects to the bottom. finished.

Picture source: Fouries on tumblr

In the field of mathematics, Klein bottle refers to a kind of unpredictable plane. In topology, Klein bottle is an unprecedented topology space. In the three -dimensional space, the structure of the Klein bottle can be expressed as: there is a hole at the bottom of a bottle, extending the neck of the bottle, and twisted into the bottle, and then connected to the bottom hole.

Unlike the cups we usually use to drink water, this object does not have "edges", and its surface will not end. Therefore, no matter where you irrigate the water, the water will flow out of this bottle, and no one can fill it. And it is different from the sphere. A bee can fly directly from the inside of the bottle to the outside without going through the surface. Therefore, there is a saying that the Klein bottle is a bottle of "never pretended".

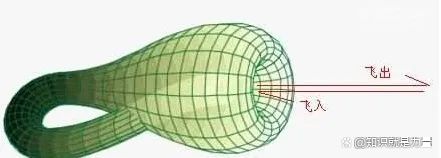
Picture source: WeChat public account "Material Studio"

Picture source: WeChat public account "Material Studio"

Theoretically, it can be constructed to construct a Klein bottle by adhesion the edges of the two Mobius Rings, but in fact it is impossible to do this in three -dimensional space (you can try). Because the bottle is a curved surface that can only be manifested in the four -dimensional space. In the three -dimensional space, we just sacrificed some characteristics and showed it as if we intersect with ourselves. In fact, its bottleneck is through the fourth -dimensional space and then connected to the bottom of the bottle, and does not pass through the bottle wall. Therefore, until now, Klein bottle is still the "fictional thing" in the mind of Felix Klein (German mathematician who discovered and named his name in 1882 and named after his name.

However, some scientists have a big brain and linked the structure of the Klein bottle with the boundary of the universe. Watching the universe is a large Klein bottle? The reason why humans have no way to reach the margin of the universe is because the universe has no margins at all, and there is no internal and external differences, which is a continuous circulation space. Therefore, no matter how advanced human technology is, it is impossible to explore the margin of the universe. No matter how far away, you can only go back to the origin. Perhaps humans want to explore the unclean mystery of the universe, and they need to create a real Klein bottle, unlock the mystery of the four -dimensional space, and enter a higher -dimensional space to unveil the veil of the universe.

In fact, whether it is the Mobius belt or the Klein bottle, it is actually a problem in the field of topology.

Topology is a discipline that studies geometric graphics or space after continuous changes, and can also maintain some unchanged disciplines. It is one of the main areas of modern mathematics. In topology, people only consider the location relationship between objects, and do not consider their shapes and sizes.

You must have heard that coffee cups and donuts are the same type of expression. In fact, in the eyes of mathematicians, coffee cups and donuts are indeed similar in essence. Why is this?

Here is a concept called the same embryo. We compare the topology to a geometric object, and the same embryo is to continuously extend and bend the object to make it a new object. Because coffee cups and donuts have a "hole" structure, a soft and soft tattoo, you can imagine it as a rubber puree, you can definitely pinch the shape of the coffee cup.

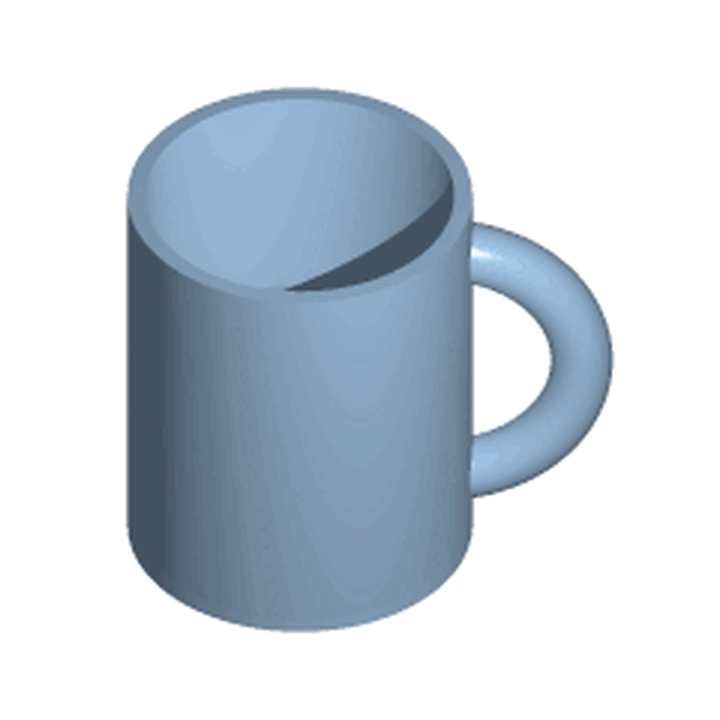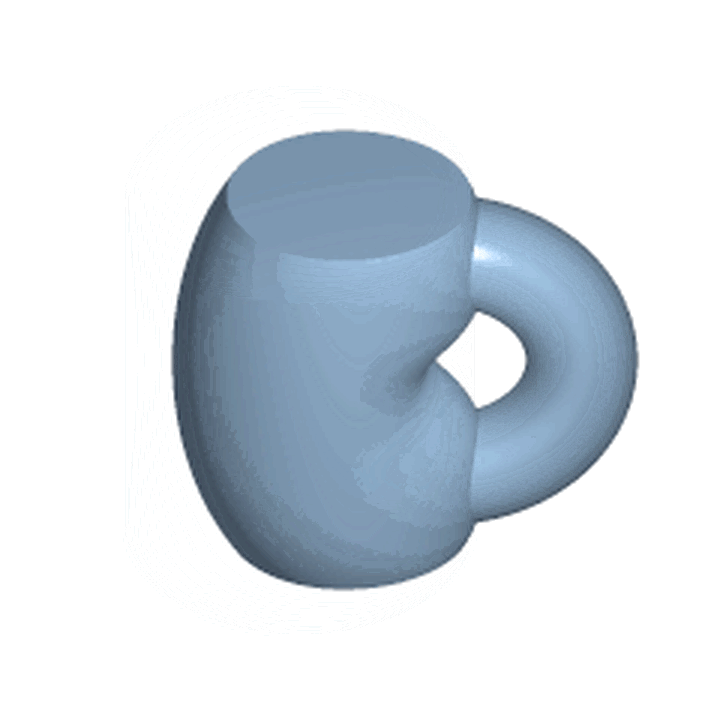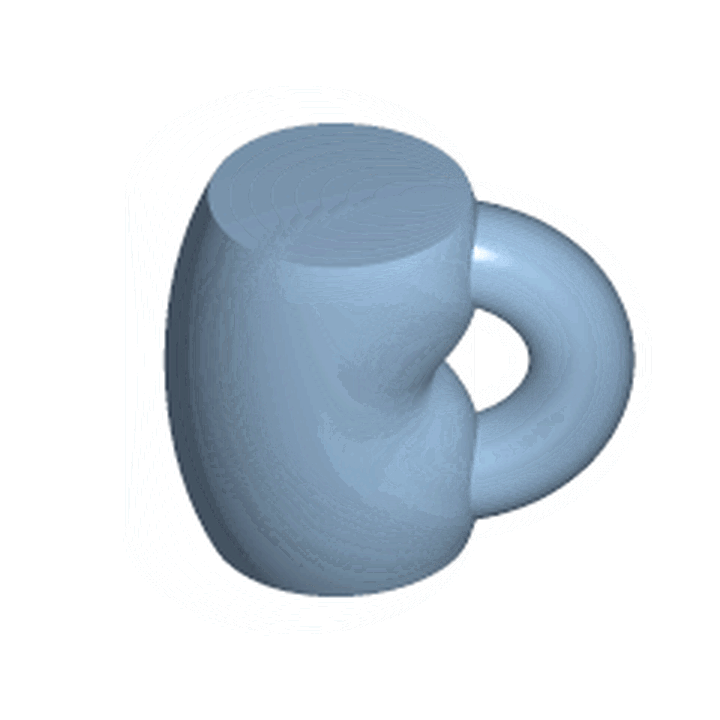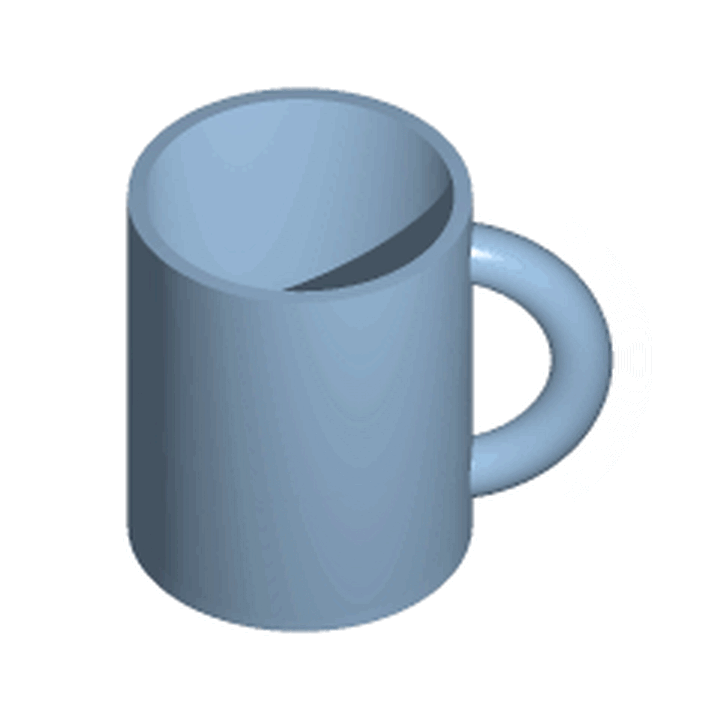
Famous coffee cups and donut animation | Wiki

However, there are some rules for pinching games:

1. Do not allow holes on the rubber puree;

2. Two points on the rubber puree are not allowed to be pinched together (we can't make the spherical rubber puree into the shape of a donut).

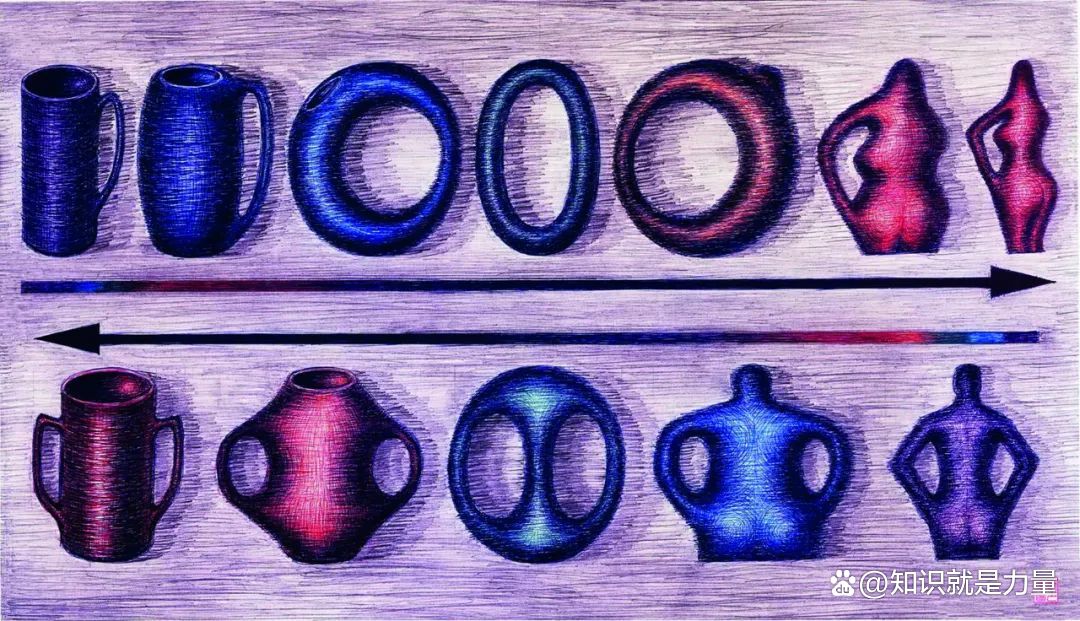
When we say that the two objects have the same topology or topology (coffee cups and donuts have the same topology), which means that even if these two objects are different in geometric shapes, they are completely on topology. equivalence. We can stretch the rubber puree into any strange shape that can be imagined, but in the world of topology, all these shapes are exactly the same.

For example, the shape of the round and square and triangular shape, although the size is different, but under the topology transformation, they are equivalent to graphics; football and football are equivalent -from the perspective of topology, their topology structure is completely completely complete Same. The surface of the swimming ring and the surface of the football have different topological properties, such as a hole in the middle of the swimming rim. In topology, the space represented by football is called the spherical side, and the space represented by the swimming ring is called the ring. The sphere and the ring are different topological spaces.

"Change of Top Turks": Regardless of the single and double handles, men and women should be equivalent to the price, single -hole and double holes are not changed. Liu Xiqing's "When Painting Injects Scientific Elements") "Peace Topping": Static and ideal dynamics, peacekeeping topology in the world, olive branches become peace pigeons. In the March issue, Liu Xiqing's "When Painting Injects Scientific Elements")

These are the topology of the three -dimensional space visualization, but an advantage of the topology is that it allows us to use the same method to describe the objects in the four -dimensional existence. It is our aforementioned Mobius ring and Klein bottle.

In fact, in our lives, there are many applications of topology. For example, the designer made the conveyor belt into the shape of the Mobius ring, which can effectively slow down the aging of the conveyor belt; Architectural design will also be used for topology.

The topology interpretation of the City of Dreams Macau, there are 3 ring handles on the surface of the building (drawing: Gu Qifeng, source: "Knowledge is Power" magazine in September 2020 "Geometry of Beijing Daxing International Airport")

Of course, we only explained the tip of the iceberg of topology. There are many branches of topology: points for topology, algebraneus topology, micro -score topology, and geometric topology. As a very important area in mathematics, it not only promotes discipline progress, but also represents the charm of mathematics.

The charm of mathematics is more than the magic of the Mobius ring and Clein bottle!

The iconic six -pointed star shape of Beijing Daxing International Airport, which is the first of the "New World's Seven Miracles", is a bold application of Mathematics Riemann Geometry in architecture; seemingly simple graphics, but formed Pueros inlaid with ever -changing patterns; The direction of the ball, the shot on the football field and the goalkeeper, the jumping point of the jump, the racing theory and throwing technology are closely related to mathematics ...

These issues, "Knowledge is Power" magazine

Reveal for you!

Not only that, these charming mathematics problems can be used as the "comprehensive and practical" learning theme of 2022 mathematics curriculum standards, but also as the theme of "cross -disciplinary theme learning" (sports and mathematics, music and mathematics, painting and mathematics, architecture and mathematics Wait), and the cultivation of the core literacy of the 2022 new mathematics curriculum standard: I will observe the real world with the eyes of mathematics, think of the real world with mathematics thinking, express the real world in the language of mathematics, and 11 core concepts: numbers A sense of sense, quantity, symbolic consciousness, reasoning consciousness, data awareness, model consciousness, application consciousness, application consciousness, innovation awareness, computing ability, geometric intuitive, space concept.

The mathematics in the magazine of "Knowledge is Power" is no longer just a boring and simple digital operation. Instead, it is the soul that gives architecture elegant forms, the notes of sensuality and rational blend of music in music, and a tour that has endless scenes ...

See the literature:

[1] Liu Xiqing. When painting is injected with scientific elements [J]. Knowledge is power, 2015, No.508 (03): 62-65.

[2] Gu Qifeng. Geometric Exploration of Beijing Daxing International Airport [J]. Knowledge is power, 2020, No.574 (09): 6-9.

[3] Deng Yichao. The topology of the magic "coat" [J]. Knowledge is power, 2021, No.584 (07): 10-11.

[4] Zhu Jiyin. A experiment with you to feel unprepared space [J]. Knowledge is power, 2022.

[5] WeChat public account "Shaanxi Normal University Mu Messing Academy" January 19, 2022 article "Klein Bottle | What? Bottle that will always be dissatisfied? "

[6] https://zhuanlan.zhihu.com/p/214200642

[7] https://wiki.mbalib.com/wiki/%E6%8B%9JP

[8] Ministry of Education. Curriculum standard for compulsory education (2022 edition) [s] .2022.

- END -